Between meetings there will be interesting problems to think about. They are meant to help grow and challenge you. Each problem will be talked about at the next meeting. Happy Solving!
Problem 3
While traveling in the Kingdom of Arbitraria, you are accused of a heinous crime. Arbitraria decides who’s guilty or innocent not through a court system, but a board game. It’s played on a simple board: a track with sequential spaces numbered from 0 to 1,000. The zero space is marked “start,” and your token is placed on it. You are handed a fair six-sided die and three coins. You are allowed to place the coins on three different (nonzero) spaces. Once placed, the coins may not be moved.
After placing the three coins, you roll the die and move your token forward the appropriate number of spaces. If, after moving the token, it lands on a space with a coin on it, you are freed. If not, you roll again and continue moving forward. If your token passes all three coins without landing on one, you are executed. On which three spaces should you place the coins to maximize your chances of survival?
After placing the three coins, you roll the die and move your token forward the appropriate number of spaces. If, after moving the token, it lands on a space with a coin on it, you are freed. If not, you roll again and continue moving forward. If your token passes all three coins without landing on one, you are executed. On which three spaces should you place the coins to maximize your chances of survival?
Problem 2
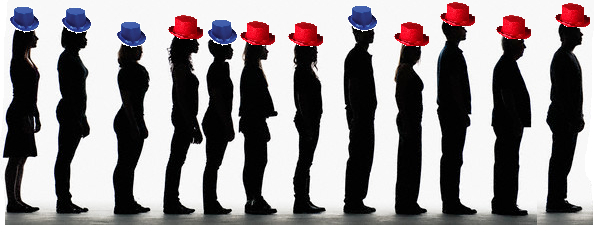
There are n=12 people in a room and each person is wearing either a blue hat or a red hat. They are standing in a line face-to-back, so that each person can see every person's hat in front of them in line, but cannot see anyone's hat behind them in line. No one can see their own hat. No information is known about how many red hats there are and how many blue hats there are.
They are going to each guess the color of their own hat, starting with the person at the back of the line, and proceeding forward one person at a time. For example, the person at the back of the line might say "blue" and the person directly in front of her might say "red". During the guessing, absolutely no communication of any kind is permitted between the people except for the stating of the words "blue" and "red", and each person may say only one of those two words.
Their goal is to devise a strategy to guarantee that at least n-1=11 of them are correct in their hat color guesses. They are permitted to talk to each other to devise this strategy before they put their hats on and begin guessing, but once the guessing starts, absolutely no communication of any kind is permitted except for the stating of the words "blue" and "red" -one word per person.
What should their strategy be?
They are going to each guess the color of their own hat, starting with the person at the back of the line, and proceeding forward one person at a time. For example, the person at the back of the line might say "blue" and the person directly in front of her might say "red". During the guessing, absolutely no communication of any kind is permitted between the people except for the stating of the words "blue" and "red", and each person may say only one of those two words.
Their goal is to devise a strategy to guarantee that at least n-1=11 of them are correct in their hat color guesses. They are permitted to talk to each other to devise this strategy before they put their hats on and begin guessing, but once the guessing starts, absolutely no communication of any kind is permitted except for the stating of the words "blue" and "red" -one word per person.
What should their strategy be?
Problem 1
A palindrome is an integer which reads the same from right to left as it does from left to right.
Example: 346,757,643 is a palindrome.
What positive integer (other than 1) divides every four-digit palindrome?
Find a justification that isn’t simply checking your answer against every possible four-digit palindrome.
Example: 346,757,643 is a palindrome.
What positive integer (other than 1) divides every four-digit palindrome?
Find a justification that isn’t simply checking your answer against every possible four-digit palindrome.
